The effect on how much longer s spring gets when hanging masses from it
An experiment was carried out where a spring was clamped vertically and its length was measured without any masses hanging from it. Masses were then added and the new length of the spring was measured. The original length of the spring was subtraced from each of these results in order to see how much longer each mass made the spring. The results were plotted on a graph.
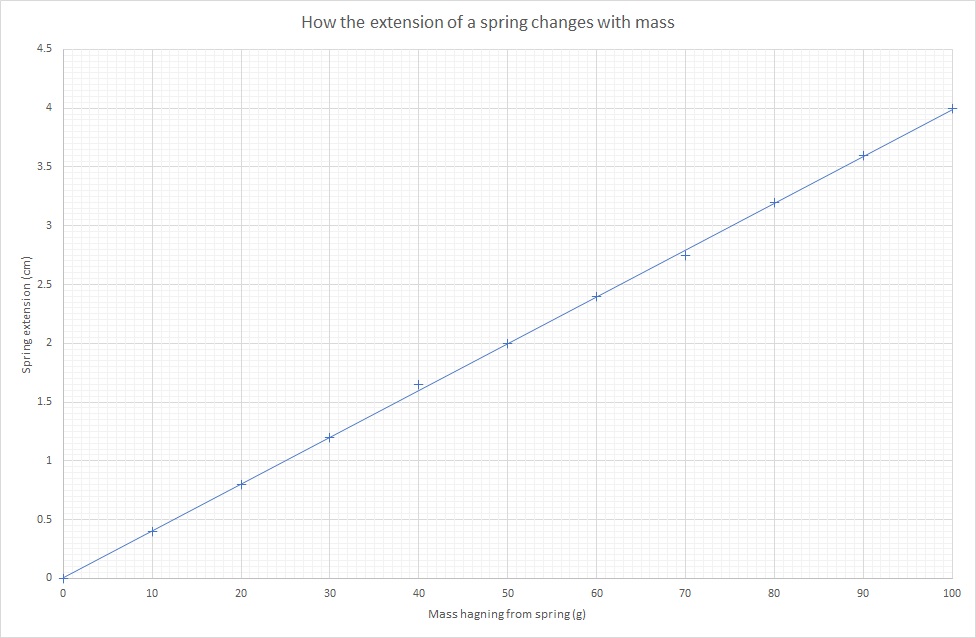
It can bee seen from the graph that the extension of the spring is directly proportional to the mass haning from it. This was shown by Robert Hooke in 1660 and is now called Hooke's Law. The relationship can be expressed as F = kx, where F is the weight force of the mass, k is the spring constant and x is the amount that the spring has been extended. This only works for small extensions of the spring.