Higher Physics

Collisions, explosions and impulse

Momentum and collisions

Momentum is a vector quantity which is always conserved in the absence of external forces.
What this means is that the total momentum of a system in the absence of external forces will be the same before any collision and after it.
When answering examination questions it is vital to remember that we have to state the TOTAL momentum of all objects before collision will be equal to the TOTAL momentum of all objects after collsion in the ABSENCE of external forces.

Momentum is calculated using the following equation: $$p=\mathrm{mv}$$ This tells us the momentum is the prouct of mass and velocity.

Example

A ball of mass 200 g moving at 4 ms^-1 collides with a stationary ball of mass 100 g. The ball that was hit moves with a velocity of 3 ms^-1. What is the velocity of the 200 g ball after the collision?
Conservation of momentum can be applied as there are no external forces.
Total momentum before collision can be calculated for each ball and then added together.
Momentum of 200 g ball: $$p=\mathrm{mv}$$ $$p=\mathrm{0.2\; x\; 4}$$ $$p={\mathrm{0.8\; kgms}}^{\mathrm{-1}}$$ Momentum of 100 g ball: $$p=\mathrm{mv}$$ $$p=\mathrm{0.1}\times 0$$ $$p={\mathrm{0\; kgms}}^{\mathrm{-1}}$$ Total mmomentum is therefore:
$$p_{\mathrm{total}}=\mathrm{0.8\; +\; 0}={\mathrm{0.8\; kgms}}^{\mathrm{-1}}$$ After the collision we know the velocity of one ball, but not the other. Since we already know the total momentum it is possible to calculate the unknown velocity.
Momentum of the 100 g ball after collision $$p=\mathrm{0.1}\times \mathrm{0.3}={\mathrm{0.3\; kgms}}^{\mathrm{-1}}$$ Momentum of 200 g ball after collision $$p=p_{\mathrm{total}}-p_{\mathrm{100}}$$ $$p=\mathrm{0.8}-\mathrm{0.3}={\mathrm{0.5\; kgms}}^{\mathrm{-1}}$$ Now we know the momentum of the 200 g ball we can work out its velocity. $$p=\mathrm{mv}$$ $$\mathrm{0.5}=\mathrm{0.2}\times v$$ $$v={\mathrm{2.5\; ms}}^{\mathrm{-1}}$$

---

Determining the speed of the ball

Attempt to solve the question yourself before clicking the solve button!

A moving ball collides with a stationary ball on a smooth level surface. The mass of the moving ball was kg and of the stationary ball it was kg. the moving ball hit the stationary ball when it was moving at a speed of m s^-1. Immediately after the collision the moving ball has only 10% of its initial velocity.
Calculate the speed of the stationary ball immediately after it has been hit.

---

Elastic Collisions

When a collision occurs the kinetic energy of the objects can be conserved. Where this occurs we have a collision that is said to be perfectly elastic. An example of such a collision is given below.

Elastic Collision Example

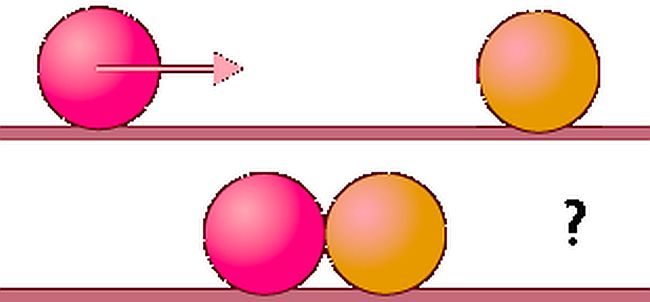
A billiard ball of mass 155 g collides with a sationary ball with the same mass. The moving ball has an initial velocity of 2.5 m/s. After collision this ball is stationary. The ball that was hit by the moving ball is now moving at 2.5 m/s. Show that the momentum is conserved and that the collision is elastic.

Since the question uses the command word "show" we must begin our answer by using the appropriate equation. $$p=\mathrm{mv}$$

and $$p_{\mathrm{total}}=m_1{v}_1+m_2{v}_2$$ When we show that the total momentum before collision is the same as the total momentum after collision the first part of the question will have been answered.

Before Collision $$p_{\mathrm{total}}=\mathrm{0.155\; \times \; 2.5}+\mathrm{0.155\; \times \; 0}$$ $$p_{\mathrm{total}}=\mathrm{0.388\; kg\; m/s}$$

After Collision $$p_{\mathrm{total}}=\mathrm{0.155\; \times \; 0}+\mathrm{0.155\; \times \; 2.5}$$ $$p_{\mathrm{total}}=\mathrm{0.388\; kg\; m/s}$$

Since these values are the same we have shown that the momentum has been conserved.

To show that the collision is elastic we need to determine if the kinetic energy in the system before the collision is the same as the kinetic energy in the system after the collision. Since the command term show has been used we must begin the answer with the appropriate equation. $$E_k=\frac{1}{2}{\mathrm{mv}}^2$$ And we should also write the equation for total kinetic energy in the system $$E_{\mathrm{k total}}=E_{\mathrm{k 1}}+E_{\mathrm{k 2}}$$ Before Collision $$E_{\mathrm{k total}}=\frac{1}{2}{m}_1{v}_1^2+\frac{1}{2}{m}_2{v}_2^2$$ $$E_{\mathrm{k total}}=\frac{1}{2}{\mathrm{\times \; 0.155\; \times \; 2.5}}^2+\frac{1}{2}{\mathrm{\times \; 0.155\; \times \; 0}}^2$$ $$E_{\mathrm{k total}}=\mathrm{0.969\; +\; 0}=\mathrm{0.969\; J}$$ After Collision $$E_{\mathrm{k total}}=\frac{1}{2}{m}_1{v}_1^2+\frac{1}{2}{m}_2{v}_2^2$$ $$E_{\mathrm{k total}}=\frac{1}{2}{\mathrm{\times \; 0.155\times \; 0}}^2+\frac{1}{2}{\mathrm{\times \; 0.155\times \; 2.5}}^2$$ $$E_{\mathrm{k total}}=\mathrm{0\; +\; 0.969}=\mathrm{0.969\; J}$$

From this we can see that the kinetic energy before the collision is the same as the kinetic energy after the collision. In the absence of any external forces this means that this is an elastic collision.

Inelastic Collision

When a collision occurs and the kinetic energy of the objects is converted to an energy other than kinetic this is said to be an inelastic collision. An example of such a collision is given below.

Two cars are moving in opposite directions. The car moving to the right has a mass of 1500 kg and is travelling at 5 m/s. The car moving to the left has a mass of 2000 kg and is moving at 3 m/s.
The cars stick to each other in the collision and move as a combined object. What speed and direction are the cars moving in after the collision? Show that this is an inelastic collision.

To solve the first part of the question we need to remember that in the absence of external forces the momentum after the collision will equal the momentum before the collision. $$P_{\mathrm{before}}=P_{\mathrm{after}}$$ $$P_{\mathrm{before}}=m_1{v}_1+m_2{v}_2$$ $$P_{\mathrm{after}}=\left(m_1+m_2\right)v_f$$ $$P_{\mathrm{before}}=\mathrm{1500\times 5}+\mathrm{2000\times (-3)}$$ $$P_{\mathrm{before}}=\mathrm{7500}+\mathrm{(-6000)}$$ $$P_{\mathrm{before}}={\mathrm{1500\; kg\; ms}}^{-1}$$

Now consider the after situation:

$$P_{\mathrm{after}}={\mathrm{1500\; kg\; ms}}^{-1}=\left(m_1+m_2\right)v_f$$ $${\mathrm{1500\; kg\; ms}}^{-1}=\left(\mathrm{1500}+\mathrm{2000}\right)v_f$$ $$v_f=\frac{1500}{3500}={\mathrm{0.43\; ms}}^{-1}$$

This value is positive, so that cars move to the right at 0.43 ms^-1 immediately after the collision.

To show that this is an inelastic collision we need to work out the total kinetic energy before the the collision, and the total kinetic energy after the collision. An inelastic collision will result in a smaller kinetic energy after the collision.

Because the question states that we must SHOW we must first write the equation that we will use.

Before Collision $$E_{\mathrm{k total}}=\frac{1}{2}{m}_1{v}_1^2+\frac{1}{2}{m}_2{v}_2^2$$ $$E_{\mathrm{k total}}=\frac{1}{2}{\mathrm{\times 1500\times 5}}^2+\frac{1}{2}{\mathrm{\times 2000\times 3}}^2$$ $$E_{\mathrm{k total}}=\mathrm{37500+18000}=\mathrm{55500\; J}$$ After Collision $$E_{\mathrm{k final}}=\left(m_1+m_2\right)v_f^2$$ $$E_{\mathrm{k final}}=\left(\mathrm{1500}+\mathrm{2000}\right)\times {0.43}^2$$ $$E_{\mathrm{k total}}=\mathrm{277\; J}$$

Notice how much kinetic energy has been "lost". You should understand that this has been transferred to the deformation of the cars, heat and sound in the collision.

---

Elastic or inelastic

Attempt to solve the question yourself before clicking the solve button!

A moving ball collides with a stationary ball on a smooth level surface. The mass of the moving ball was kg and of the stationary ball it was kg. the moving ball hit the stationary ball when it was moving at a speed of m s^-1. Immediately after the collision the moving ball has only % of its initial velocity.
(a) Determine the total momentum of the system.
(b) Calculate the speed of the stationary ball immediately after it has been hit.
(c) Calculate the kinetic energy before the collision and after the collision
(d) Using your results from part (c) state if the collision is elastic or inelastic, with your reason.

---

Recoiling Collision

Attempt to solve the question yourself before clicking the solve button!

A moving ball collides head on with a second ball moving in the opposite direction on a smooth level surface. The mass of the first moving ball was kg and of the second ball moving in the opposite direction it was kg. The first moving ball was moving right at a speed of m s^-1. The second ball was moving left at a speed of m s^-1 Immediately after the collision the first moving ball moves to the left with a speed of m s^-1.
Calculate the speed of the second ball immediately after the collision.

---

Inelastic Collision

Attempt to solve the question yourself before clicking the solve button!

A toy train engine with a mass of g collides with a wagon of mass g. The train engine and wagon are both moving in the same direction at the time of the collision. Their respective speeds are m s^-1 and m s^-1. The train couples with the wagon and they continue moving together.
(a) Determine the speed of the train and wagon immediately after the collision.
(b) Show by calculation that this is an inelastic collision.

---

Linear Momentum and Collisions PowerPoint

Elastic and Inelastic Collisions

Force and Impulse